1. Four particles A, B, C and D having masses m, 2m, 3m and 4m respectively are placed in order at the corners of a square of side a. Locate the centre of mass.

2. Two identical uniform rods AB and CD, each of length L are jointed to form a T-shaped frame as shown in figure. Locate the centre of mass of the frame. The centre of mass of a uniform rod is at the middle point of the rod.
Sol. Let the mass of each rod be m. Take the centre C of the rod AB as the origin and CD as the Y-axis. The rod AB has mass m and its centre of mass is at C. For the calculation of the centre of mass of the combined system, AB may be replaced by a point particle of mass m placed at the point C. Similarly the rod CD may be replaced by a point particle of mass m placed at the centre E of the rod CD. Thus, the frame is equivalent to a system of two particles of equal masses m each, placed at C and E. The centre of mass of this pair of particles will be at the middle point F of CE.
The centre of mass of the frame is, therefore, on the rod CD at a distance L/4 from C.
3. Two charged particles of masses m and 2m are placed a distance d apart on a smooth horizontal table. Because of their mutual attraction, they move towards each other and collide. Where will the collision occur with respect to the initial positions?
Sol. As the table is smooth, there is no friction. The weight of the particles and the normal force balance each other as there is no motion in the vertical direction. Thus, taking the two particles as constituting the system, the sum of the external forces acting on the system is zero. The forces of attraction between the particles are the internal forces as we have included both the particles in the system. Therefore, the centre of mass of the system will have no acceleration.
Initially, the two particles are placed on the table and their velocities are zero. The velocity of the centre of mass is, therefore, zero. As time passes, the particles move, but the cenre of mass will continue to be at the same place. At the time of collision, the two particles are at one place and the centre of mass will also be at that place. As the centre of mass does not move, the collision will take place at the centre of mass.
The centre of mass will be at a distance 2d/3 from the initial position of the particle of mass m towards the other particle and the collision will take place there.
4. Each of the blocks shown in figure has mass 1 kg. The rear block moves with a speed of
2 m/s towards the front block kept at rest. The spring attached to the front block is light and has a spring constant 50 N/m. Find the maximum compression of the spring.
![]()
Sol. Maximum compression will take place when the blocks move with equal velocity. As no net external force acts on the system of the two blocks, the total linear momentum will remain constant. If V is the common speed at maximum compression, we have,
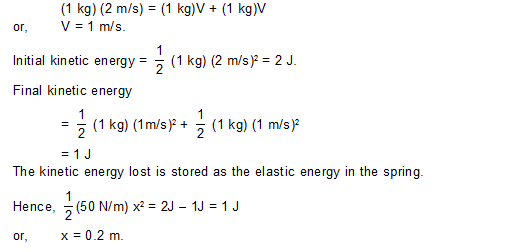
5. A cart A of mass 50 kg moving at a speed of 20 km/h hits a lighter cart B of mass 20 kg moving towards it at a speed of 10 km/h. The two carts cling to each other. Find the speed of the combined mass after the collision.
Sol. This is an example of inelastic collision. As the carts move towards each other, their momenta have opposite sign. If the common speed after the collision is V, momentum conservation gives
(50 kg) (20 km/h) – (20 kg) (10 km/h) = (70 kg)V
or V = 80/7 km/h.
6. A block of mass m moving at speed v collides with another block of mass 2 m at rest. The lighter block comes to rest after the collision. Find the coefficient of restitution.
Sol. Suppose the second block moves at speed v¢ after the collision. From the principle of conservation of momentum,
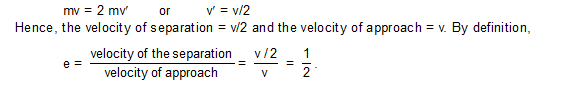
1. Can the centre of mass of a body be at a point outside the body ?
2. If all the particles of a system lie in X-Y plane, is it necessary that the centre of mass be in X-Y plane ?
3. If all the particle of a system lie in a cube, is it necessary that the centre of mass be in the cube?
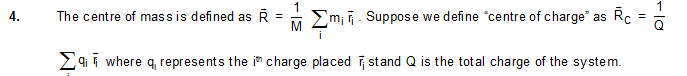
(a) can the centre of charge of a two-charge system be outside the line segment joining the charges.
(b) If all the charges of a system are in X-Y plane, is it necessary that the centre of charge be in X-Y plane ?
(c) If all the charges of a system lie in a cube, is it necessary that the centre of charge be in the cube ?
5. The weight Mg of an extended body is generally shown in a diagram to act through the centre of mass. Does it mean that the earth does not attract other particles ?
6. A bob suspended from the ceiling of a car which is accelerating on a horizontal road. The bob stays at rest with respect to the car with the string making an angle q with the vertical. The linear momentum of the bob as seen from the road is increasing with time. Is it a violation of conservation of linear momentum? If not, where is the external force which changes the linear momentum?
7. You are waiting for a train on a railway platform. Your three year old niece is standing on your iron trunck containing the luggage. Why does the trunck not recoil as she jumps off on the platform?
8. In a head-on collision between two particles, is it necessary that the particles will acquire a common velocity at least for one instant?
9. A collision experiment is done on a horizontal table kept in an elevator. Do you expect a change in the results if the elevator is accelerated up or down because of the non-inertial character of the frame?
10. Two bodies make an elastic head - on collision on a smooth horizontal table kept in a car. Do you expect a change in the result if the car is accelerated on a horizontal road because of the non-inertial character of the frame? Does the equation “Velocity of separation = Velocity of approach” remain valid in an accelerating car? Does the equation “final momentum = initial momentum” remain valid in the accelerating car ?
11. If the total mechanical energy of a particle is zero, is its linear momentum necessarily zero ? Is it necessarily nonzero ?
12. If the linear momentum of a particle is known, can you find its kinetic energy ? If the kinetic energy of a particle is known, can you find its linear momentum?
13. What can be said about the centre of mass of a uniform hemisphere without making any calculation? Will its distance from the centre be more than r/2 or less than r/2?
14. You are holding a cage containing a bird. Do you have to make less effort if the bird flies from its position in the cage and manages to stay in the middle without touching the walls of the cage? Does it make a difference whether the cage is completely closed or it has rods to let air pass?
15. A fat person is standing on a light plank floating on a calm lake. The person walks from one end to the other on the plank. His friend sitting on the shore watches him and finds that the person hardly moves any distance because the plank moves backward about the same distance as the person moves on the plank. Explain.
16. A high - jumper successfully clears the bar. Is it possible that his centre of mass crossed the bar from below it. Try it with appropriate figures.
17. Which of the two persons shown in figure is more likely to fall down? Which external force is responsible for his falling down?

18. Suppose we define a quantity ‘Linear Pomentum’ as linear pomentum = mass × speed.
The linear pomentum of a system of particles is the sum of linear pomenta of the individual particles. Can we state a principle of conservation of linear pomentum as “linear pomentum of a system remains constant if no external force acts on it”?
19. Use the definition of linear pomentum from the previous question. Can we state the principle of conservation of linear pomentum for a single particle?
20. To accelerate a car we ignite petrol in the engine of the car. Since only an external force can accelerate the centre of mass, is it proper to say that “the force generated by the engine accelerates the car”?
21. A ball is moved on a horizontal table with some velocity. The ball stops after moving some distance. Which external force is responsible for the change in the momentum of the ball?
22. Consider the situation of the previous problem. Take “the table plus the ball” as the system. Friction between the table and the ball is then an internal force. As the ball slows down, the momentum of the system decreases. Which external force is responsible for this change in the momentum?
23. When a nucleus at rest emits a beta particle, it is found that the velocities of the recoiling nucleus and the beta particle are not along the same straight line. How can this be possible in view of the principle of conservation of momentum?
24. A van is standing on a frictionless portion of a horizontal road. To start the engine, the vehicle must be set in motion in the forward direction. How can the persons sitting inside the van do it without coming out and pushing from behind?
25. In one - dimensional elastic collision of equal masses, the velocities are interchanged. Can velocities in a one dimensional collision be interchanged if the masses are not equal ?
1. Consider the following two equations
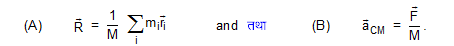
In a non-inertial frame
(A) both are correct (B) both are wrong
(C*) A is correct but B is wrong (D) B is correct but A is wrong
2. Consider the following two statements :
(a) linear momentum of the system remains constant
(b) centre of mass of the system remains at rest.
(A) a implies b and b implies a
(B) a does not imply b and b does not imply a
(C) a implies b but b does not imply a
(D*) b implies a but a does not imply b
3. Consider the following two statements :
(a) Linear momentum of the system of particles is zero
(b) Kinetic energy of a system of particles is zero
(A) a implies b and b implies a
(B) a does not imply b and b does not imply a
(C) a implies b but b does not imply a
(D*) b implies a but a does not imply a
4. Consider the following two statements :
(a) the linear momentum of a particle is independent of the frame of reference
(b) the kinetic energy of a particle is independent of the frame of reference
(A) both a and b are true (B) a is true but b is false
(C) a is false but b is true (D*) both a and b are false
5. All the particles of a body are situated at a distance R from the origin. The distance of the centre of mass of the body from the origin is
(A) = R (B*) £ R (C*) > R (D) ³ R
6. A circular plate of diameter d is kept in contact with a square plate of edge d as shown in figure. The density of the material and the thickness are same everywhere. The centre of mass of the composite system will be

(A) inside the circular plate (B) inside the square plate
(C) at the point of contact (D*) outside the system
7. Consider a system of two identical particles. One of the particles is at rest and the other has an acceleration . The centre of mass has an acceleration.

8. Internal forces can change
(A) the linear momentum but not the kinetic energy
(B*) the kinetic energy but not the linear momentum
(C) linear momentum as well as kinetic energy
(D) neither the linear momentum nor the kinetic energy
9. A bullet hits a block kept at rest on a smooth horizontal surface and gets embedded into it. Which of the following does not change ?
(A) linear momentum of the block (B) kinetic energy of the block
(C*) gravitational potential energy of the block (D) temperature of the block
10. A uniform sphere is placed on a smooth horizontal surface and a horizontal force F is applied on it at a distance h above the surface. The acceleration of the centre
(A) is maximum when h = 0 (B) is maximum when h = R
(C) is maximum when h = 2R (D*) is independent of h
11. A body falling vertically downwards under gravity breaks in two parts of unequal masses. The centre of mass of the two parts taken together shifts horizontally towards
(A) heavier piece
(B) lighter piece
(C*) does not shift horizontally
(D) depends on the vertical velocity at the time of breaking
12. A ball kept in a closed box moves in the box making collisions with the walls. The box is kept on a smooth surface. The velocity of the centre of mass
(A) of the box remains constant
(B*) of the box plus the ball system remains constant
(C) of the ball remains constant
(D) of the ball relative to the box remains constant
13. A body at rest breaks into two pieces of equal masses. The parts will move
(A) in same direction (B) along different lines
(C*) in opposite directions with equal speeds (D) in opposite directions with unequal speeds
14. A heavy ring of mass m is clamped on the periphery of a light circular disc. A small particle having equal mass is clamped at the centre of the disc. The system is rotated in such a way that the centre moves in a circle of radius r with a uniform speed v. We conclude that an external force

15. The quantities remaining constant in a collision are
(A) momentum, kinetic energy and temperature
(B) momentum and kinetic energy but not temperature
(C) momentum and temperature but not kinetic energy
(D*) momentum, but neither kinetic energy nor temperature

17. A shell is fired from a canon with a velocity V at an angle q with the horizontal direction. At the highest point in its path, it explodes into two pieces of equal masses. One of the pieces retraces it path to the cannon. The speed of the other piece immediately after the explosion is

18. In an elastic collision
(A*) the initial kinetic energy is equal to the final kinetic energy
(B) the final kinetic energy is less than the initial kinetic energy
(C) the kinetic energy remains constant
(D) the kinetic energy first increases then decreases.
19. In an inelastic collision
(A) the initial kinetic energy is equal to the final kinetic energy
(B*) the final kinetic energy is less than the initial kinetic energy
(C) the kinetic energy remains the constant
(D) the kinetic energy first increases then decreases
1. The centre of mass of a system of particles is at the origin. It follows that
(A) the number of particles to the right of the origin is equal to the number of particles to the left
(B) the total mass of the particles to the right of the origin is same as the total mass to the left of the origin
(C) the number of particles on X-axis should be equal to the number of particles on Y-axis.
(D) if there is a particle on the positive X-axis, there must be at least one particle on the negative X-axis.
Ans. None
2. A body has its centre of mass at the origin. The x-coordinates of the particles
(A) may be all positive
(B) may be all negative
(C*) may be all non-negative
(D*) may be positive for some cases and negative in other cases
3. In which of the following cases the centre of mass of a rod is certainly not at its centre ?
(A*) the density continuously increases from left to right
(B*) the density continuously decreases from left to right
(C) the density decreases from left to right upto the centre and then increases
(D) the density increases from left to right upto the centre and then decreases
4. If the external forces acting on a system have zero resultant, the centre of mass
(A) must not move (B*) must not accelerate
(C*) may move (D) may accelerate
5. A nonzero external force acts on a system of particles. The velocity and the acceleration of the centre of mass are found to be v0 and a0 at an instant t. It is possible that
![]()
6. Two balls are thrown simultaneously in air. The acceleration of the centre of mass of the two balls while in air
(A) depends on the direction of the motion of the balls
(B) depends on the masses of the two balls
(C) depends on the speeds of the two balls
(D*) is equal to g
7. A block moving in air breaks in two parts and the parts separate
(A*) the total momentum must be conserved (B) the total kinetic energy must be conserved
(C) the total momentum must change (D*) the total kinetic energy must change
8. In an elastic collision
(A) the kinetic energy remains constant
(B*) the linear momentum remains constant
(C*) the final kinetic energy is equal to the initial kinetic energy
(D*) the final linear momentum is equal to the initial linear momentum
9. A ball hits a floor and rebounds after an inelastic collision. In this case
(A) the momentum of the ball just after the collision is same as that just before the collision
(B) the mechanical energy of the ball remains the same during the collision
(C*) the total momentum of the ball and the earth is conserved
(D*) the total energy of the ball and the earth remains the same
10. A body moving towards a finite body at rest collides with it. It is possible that
(A) both the bodies come to rest
(B*) both the bodies move after collision
(C*) the moving body comes to rest and the stationary body starts moving
(D) the stationary body remains stationary, the moving body changes its velocity.
11. In head on elastic collision of two bodies of equal masses
(A*) the velocities are interchanged
(B*) the speeds are interchanged
(C*) the momenta are interchanged
(D*) the faster body slows down and the slower body speeds up.
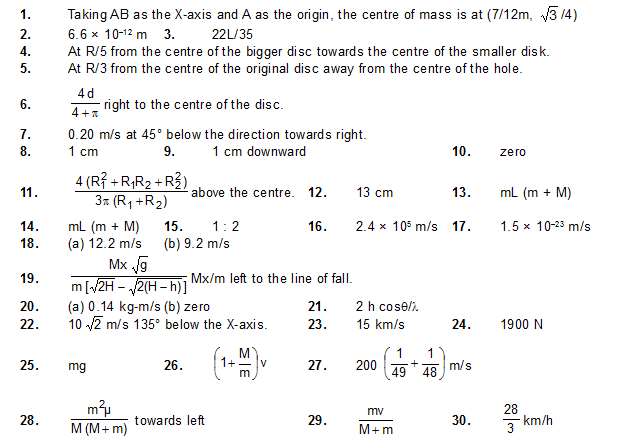
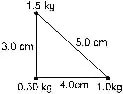
1. Three particles of masses 0.5 kg, 1.0 kg and 1.5 kg are placed at the three corners of a right angled triangle of sides 3.0 cm, 4.0 cm and 5.0 cm as shown in figure. Locate the centre of mass of the system.

2. Half of the rectangular plate shown in figure is made of a material of density r1 and the other half of density r2. The length of the plate is L. Locate the centre of mass of the plate.
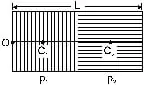
Sol. The centre of mass of each half is located at the geometrical centre of that half. Thus, the left half may be replaced by a point particle of mass Kr1 placed at C1 and the right half may be replaced by a point particle of mass Kr2 placed at C2. This replacement is for the specific purpose of locating the combined centre of mass. Take the middle point of the left edge to be the origin. The x-coordinate of C1 is L/4 and that of C2 is 3L/4. Hence, the x-coordinate of the centre of mass is

The combined centre of mass is this much to the right of the assumed origin.
3. The density of a linear rod of length L varies as r = A + Bx where x is the distance from the left end. Located the centre of mass.
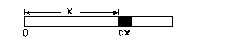
Sol. Let the cross-sectional area be a. The mass of an element of length dx located at a distance x away from the left end is (A + Bx)a dx. The x-coordinate of the centre of mass is given by
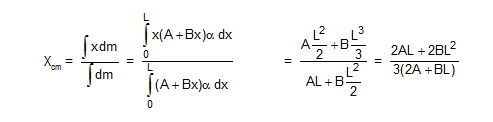
4. A cubical block of ice of mass m and edge L is placed in a large tray of mass M. If the ice melts, how far does the centre of mass of the system “ice plus tray” come down?
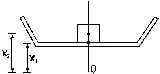
Sol. Consider figure. Suppose the centre of mass of the tray is a distance x1 above the origin and that of the ice is a distance x2 above the origin. The height of the centre of mass of the ice-tray system is
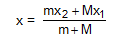
When the ice melts, the water of mass m spreads on the surface of the tray. As the tray is large, the height of water is negligible. The centre of mass of the water is then on the surface of the tray and is at a distance x2 – L/2 above the origin. The new centre of mass of the ice-tray system will be at the height
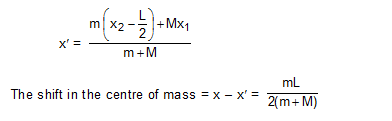
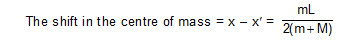
5. Consider a two-particle system with the particles having masses m1 and m2. If the first particle is pushed towards the centre of mass through a distance d, by what distance should the second particle be moved so as to keep the centre of mass at the same position?
Sol. Consider figure. Suppose the distance of m1 from the centre of mass C is x1 and that of m2 from C is x2. Suppose the mass m2 is moved through a distance d¢ towards C so as to keep the centre of mass at C.
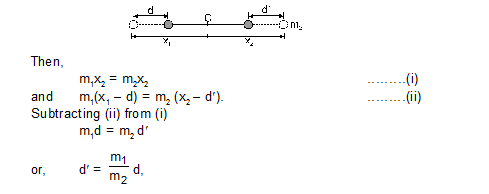
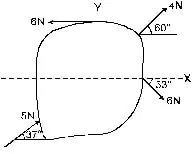
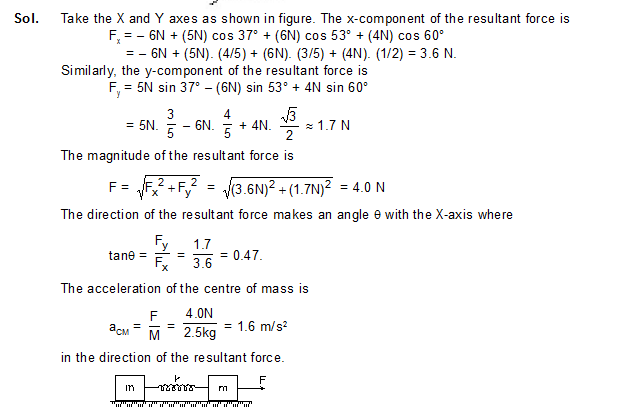
6. A body of mass 2.5 kg is subjected to the forces shown in figure. Find the acceleration of the centre of mass.
7. Two blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on one of the blocks pulling it away from the other as shown in figure. (a) Find the position of the centre of mass at time t. (b) If the extension of the spring is x0 at time t, find the displacement of the two blocks at this instant.
![]()
Sol.(a) The acceleration of the centre of mass is given by
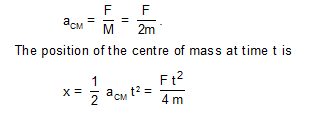
(b) Suppose the displacement of the first block is x1 and that of the second is x2. As the centre of mass is at x, we should have
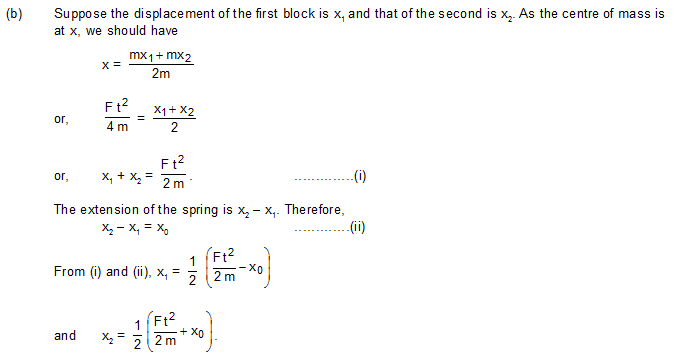
8. A projectile is fired at a speed of 100 m/s at an angle of 37° above the horizontal. At the highest point, the projectile breaks into two parts of mass ratio 1 : 3, the smaller coming to rest. Find the distance from the launching point to the point where the heavier piece lands.
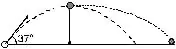
Sol. See figure. At the highest point, the projectile has horizontal velocity. The lighter part comes to rest. Hence the heavier part will move with increased horizontal velocity. In vertical direction, both parts have zero velocity and undergo same acceleration, hence they will cover equal vertical displacements in a given time. Thus, both will hit the ground together. As internal forces do not affect the motion of the centre of mass, the centre of mass hits the ground at the position where the original projectile would have landed. The range of the original projectile is

9. A block of mass M is placed on the top of a bigger block of mass 10 M as shown in figure. All the surfaces are frictionless. The system is released from rest. Find the distance moved by the bigger block at the instant the smaller block reaches the ground.
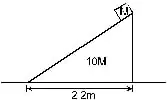
Sol. If the bigger block moves towards right by a distance X, the smaller block will move towards left by a distance (2.2 m – X). Taking the two blocks together as the system, there is no horizontal external force on it. The centre of mass, which was at rest initially, will remain at the same horizontal position.
Thus,
M (2.2 m – X) = 10 MX
or, 2.2 m = 11x
or, X = 0.2 m.
10. The hero of a stunt film fires 50 g bullets from a machine gun, each at a speed of 1.0 km/s. If he fires 20 bullets in 4 seconds, what average force does he exert against the machine gun during this period?

In order to hold the gun, the hero must exert a force of 250 N against the gun.
11. A block moving horizontally on a smooth surface with a speed of 20 m/s bursts into two equal parts continuing in the same direction. If one of the parts moves at 30 m/s, with what speed does the second part move and what is the fractional change in the kinetic energy?
= = .
12. A car of mass M is moving with a uniform velocity v on a horizontal road when the hero of a hindi film drops himself on it from above. Taking the mass of the hero to be m, what will be the velocity of the car after the event?
Sol. Consider the car plus the hero as the system. In the horizontal direction, there is no external force. Since the hero has fallen vertically, so his initial horizontal momentum = 0.
Initial horizontal momentum of the system = Mv towards right.
Finally the hero sticks to the roof of the car, so they move with equal horizontal velocity say V. Final horizontal momentum of the system.
= (M + m) V
Hence, Mv = (M + m) V
or, V = Mv/(M+m)
13. A space shuttle, while travelling at a speed of 4000 km/h with respect to the earth, disconnects and ejects a module backward, weighing one fifth of the residual part. If the shuttle ejects the disconnected module at a speed of 100 km/h with respect to the state of the shuttle before the ejection, find the final velocity of the shuttle.
Sol. Suppose the mass of the shuttle including the module is M. The mass of the module will be M/6. The total linear momentum before disconnection

14. A boy of mass 25 kg stands on a board of mass 10 kg which in turn is kept on a frictionless horizontal ice surface. The boy makes a jump with a velocity component 5 m/s in a horizontal direction with respect to the ice. With what velocity does the board recoil? With what rate are the boy and the board separating from each other ?
Sol. Consider the “board + boy” as a system. The external forces on this system are (a) weight of the system and (b) normal contact force by the ice surface. Both these forces are vertical and there is no external force in horizontal direction. The horizontal component of linear momentum of the “board + boy” system is, therefore, constant.
If the board recoils at a speed v,
0 = (25 kg) × (5 m/s) – (10 kg) v
or, v = 12.5 m/s.
The boy and the board are separating with a rate
5m/s + 12.5 m/s = 17.5 m/s.
15. A man of mass m is standing on a platform of mass M kept on smooth ice. If the man starts moving on the platform with a speed v relative to the platform, with what velocity relative to the ice does the platform recoil ?
Sol. Consider the situation shown in figure. Suppose the man moves at a speed w towards right and the platform recoils at a speed V towards left, both relative to the ice. Hence, the speed of the man relative to the platform is V + w. By the question,
V + w = v, or w = v – V .............(i)
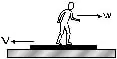
Taking the platform and the man to be the system, there is no external horizontal force on the system. The linear momentum of the system remains constant. Initially both the man and the platform were at rest. Thus,
0 = MV - mw
or, MV = m (v – V) [Using (i)]
or, V = .
16. A ball of mass m, moving with a velocity v along X-axis, strikes another ball of mass 2m kept at rest. The first ball comes to rest after collision and the other breaks into two equal pieces. One of the pieces starts moving along Y-axis with a speed v1. What will be the velocity of the other piece?
Sol. The total linear momentum of the balls before the collision is mv along the X-axis. After the collision, momentum of the first ball = 0, momentum of the first piece = mv1 along the Y-axis and momentum of the second piece = mv2 along its direction of motion where v2 is the speed of the second piece. These three should add to mv along the X-axis, which is the initial momentum of the system.
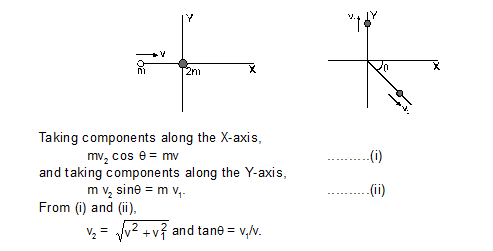
17. A bullet of mass 50 g is fired from below into the bob of mass 450 g of a long simple pendulum as shown in figure. The bullet remains inside the bob and the bob rises through a height of
1.8 m. Find the speed of the bullet. Take g = 10 m/s2.
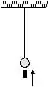
Sol. Let the speed of the bullet be v. Let the common velocity of the bullet and the bob, after the bullet is embedded into the bob, is V. By the principle of conservation of the linear momentum,
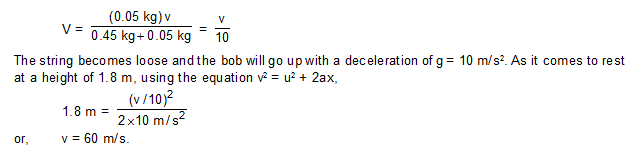
18. A light spring of spring constant k is kept compressed between two blocks of masses m and M on a smooth horizontal surface. When released, the blocks acquire velocities in opposite directions. The spring loses contact with the blocks when it acquires natural length. If the spring was initially compressed through a distance x, find the speeds of the two blocks.

Sol. Consider the two blocks plus the spring to be the system. No external force acts on this system in horizontal direction. Hence, the linear momentum will remain constant. As the spring is light, it has no linear momentum. Suppose the block of mass M moves with a speed V and the other block with a speed v after losing constant with the spring. As the blocks are released from rest, the initial momentum in zero. The final momentum is MV – mv towards right. Thus,
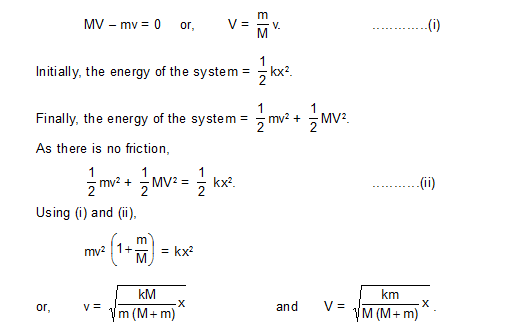
19. A block of mass m is connected to another block of mass M by a massless spring of spring constant k. The blocks are kept on a smooth horizontal plane. Initially, the blocks are at rest and the spring is unstretched when a constant force F starts acting on the block of mass M to pull it. Find the maximum extension of the spring.

Sol. Let us take the two blocks plus the spring as the system. The centre of mass of the system moves with an acceleration a = . Let us work from a reference frame with its origin at the centre of mass. As this frame is accelerated with respect to the ground we have to apply a pseudo force ma towards left on the block of mass m and Ma towards left on the block of mass M. The net external force on m is
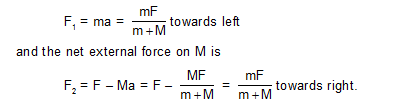
The situation from this frame is shown in figure. As the centre of mass is at rest in this frame, the blocks move in opposite directions and come to instantaneous rest at some instant. The extension of the spring will be maximum at this instant. Suppose the left block is displaced through a distance x1 and the right block through a distance x2 from the initial positions. The total work done by the external forces F1 and F2 in this period are
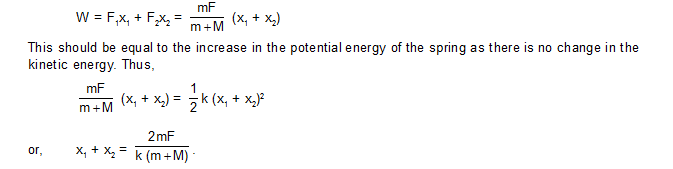
This is the maximum extension of the spring.
20. The two balls shown in figure are identical, the first moving at a speed v towards right and the second staying at rest. The wall at the extreme right is fixed. Assume all collisions to be elastic. Show that the speeds of the balls remain unchanged after all the collision have taken place.
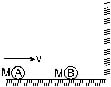
Sol. Ist collision: As the balls have equal mass and make elastic collision, the velocities are interchanged. Hence, after the first collision, the ball A comes to rest and the ball B moves towards right at a speed v.
2nd collision: The ball moving with a speed v, collides with the wall and rebounds. As the wall is rigid and may be taken to be of infinite mass, momentum conservation gives no useful result. Velocity of separation should be equal to the velocity of approach. Hence, the ball rebounds at the same speed v towards left.
3rd collision: The ball B moving towards left at the speed v again collides with the ball A kept at rest. As the masses are equal and the collision is elastic, the velocities are interchanged. Thus, the ball B comes to rest and the ball A moves towards left at a speed v. No further collision takes place. Thus, the speeds of the balls remain the same as their initial values.
21. A block of mass m moving at a speed v collides with another block of mass 2m at rest. The lighter block comes to rest after the collision. Find the coefficient of restitution.
Sol. Suppose the second block moves at a speed v' after the collision. By conservation of momentum,

22. A block of mass 1.2 kg moving at a speed of 20 cm/s collides head-on with a similar block kept at rest. The coefficient of restitution is 3/5. Find the loss of the kinetic energy during the collision.
Sol. Suppose the first block moves at a speed v1 and the second at v2 after the collision. Since the collision is head-on, the two blocks move along the original direction of motion of the first block.
23. A ball of mass m hits a floor with a speed v making an angle of incidence q with the normal. The coefficient of restitution is e. Find the speed of the reflected ball and the angle of reflection of the ball.
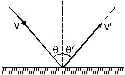
Sol. See figure. Suppose the angel of reflection is q¢ and the speed after the collision is v¢. The floor exerts a force on the ball along the normal during the collision. There is no force parallel to the surface. Thus, the parallel component of the velocity of the ball remains unchanged. This gives
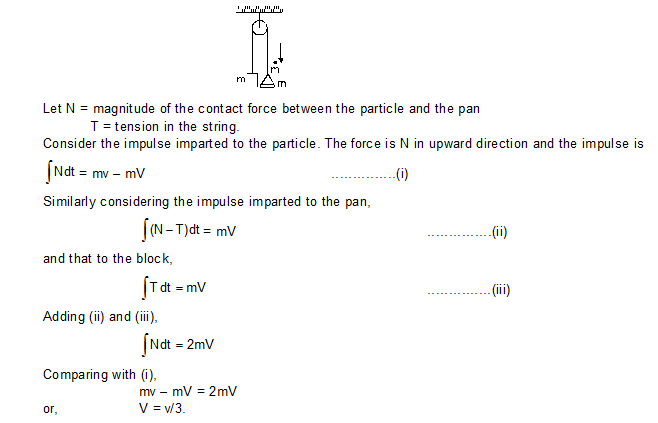
24. A block of mass m and a pan of equal mass are connected by a string going a smooth light pulley as shown in figure. Initially the system is at rest when a particle of mass m falls on the pan and sticks to it. If the particle strikes the pan with a speed v find the speed with which the system moves just after the collision.
Sol. Let the required speed be V.
As there is a sudden change in the speed of the block, the tension must change by a large amount during the collision.
1. Three particles of masses 1.0 kg, 2.0 kg and 3.0 kg are placed at the corners A, B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
Ans. Taking AB as the X-axis and A as the origin, the centre of mass is at (7/12m, /4)
2. The structure of a water molecule is shown in figure. Find the distance of the centre of mass of the molecule from the centre of the oxygen atom.
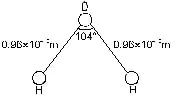
Ans. 6.6 × 10–12 m
3. Seven homogeneous bricks, each of length L, are arranged as shown in figure. Each brick is displaced with respect to the one in contact by L/10. Find the x-coordinate of the centre of mass relative to the origin shown.

Ans. 22L/35
4. A uniform disc of radius R is put over another uniform disc of radius 2R of the same thickness and density. The peripheries of the two discs touch each other. Located the centre of mass of the system.
Ans. At R/5 from the centre of the bigger disc towards the centre of the smaller disk.
5. A disc of radius R is cut out from a larger disc of radius 2R in such a way that the edge of the hole touches the edge of the disc. Locate the centre of mass of the residual disk.
Ans. At R/3 from the centre of the original disc away from the centre of the hole.
6. A square plate of edge d and a circular disc of diameter d are placed touching each other at the midpoint of an edge of the plate as shown in figure. Locate the centre of mass of the combination, assuming same mass per unit area for the two plates.
Ans. 4d/(4+pi) right to the centre of the disc.
7. Calculate the velocity of the centre of mass of the system of particle shown in figure.
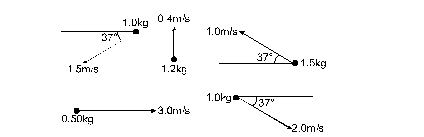
Ans. 0.20 m/s at 45° below the direction towards right.
8. Two blocks of masses 10 kg and 20 kg are placed on the x-axis. The first mass is moved on the axis by a distance of 2 cm. By what distance should the second mass be moved to keepthe position of the centre of mass unchanged?
Ans. 1 cm
9. Two blocks of masses 10 kg and 30 kg are placed along a vertical line. The first block is raised through a height of 7 cm. By what distance should the second mass be moved to raise the centre of mass by 1 cm ?
Ans. 1 cm downward
10. Consider a gravity-free hall in which a tray of mass M, carrying a cubical block of ice of mass m and edge L, is at rest in the middle (figure show). If the ice melts, by what distance does the centre of mass of “ the tray plus the ice” system descend?

Gravity free ball
Ans. zero
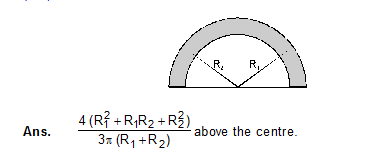
11. Find the centre of mass of a uniform plate having semicircular inner and outer boundaries of radii R1 and R2 (figure).
12. Mr. Verma (50 kg) and Mr. Mathur (60 kg) are sitting at the two extremes of a 4 m long boat (40 kg) standing still in water. To discuss a mechanics problem, they come to the middle of the boat. Neglecting friction with water, how far does the boat move in the water during the process ?
Ans. 13 cm
13. A cart of mass M is at rest on a frictionless horizontal surface and a pendulum bob of mass m hangs from the roof of the cart (figure). The string breaks, the bob falls on the floor, makes several collisions on the floor and finally lands up in a small slot made in the floor. The horizontal distance between the string and the slot is L. Find the displacement of the cart during this process.

Ans. mL (m + M)
14. The balloon, the light rope and the monkey shown in figure are at rest in the air. If the monkey reaches the top of the rope, by what distance does the balloon descend? Mass of the balloon = M, mass of the monkey = m and the length of the rope ascended by the monkey = L.

Ans. mL (m + M)
15. Find the ratio of the linear momenta of two particles of masses 1.0 kg and 4.0 kg if their kinetic energies are equal.
Ans.1 : 2
16. A uranium-238 nucleus, initially at rest, emits an alpha particle with a speed of 1.4× 107 m/s. Calculate the recoil speed of the residual nucleus thorium-234. Assume that the mass of a nucleus is proportional to the mass number.
Ans. 2.4 × 105 m/s
17. A man of mass 50 kg starts moving on the earth and acquires a speed of 1.8 m/s. With what speed does the earth recoil? Mass of earth = 6 × 1024 kg.
Ans. 1.5 × 10–23 m/s
18. A neutron initially at rest, decays into a proton, an electron and an antineutrino. The ejected electron has a momentum of 1.4 × 10–26 kg-m/s and the antineutrino 6.4 × 10–27 kg-m/s. Find the recoil speed of the proton (a) if the electron and the anitneutrino are ejected along the same direction and (b) if they are ejected along perpendicular directions. Mass of the proton = 1.67 × 10–27 kg.
Ans. (a) 12.2 m/s (b) 9.2 m/s
19. A man of mass M having a bag of mass m slips from the roof of a tall building of height H and starts falling vertically (figure). When at a height h from the ground, he notices that the ground below him is pretty hard, but there is a pond at a horizontal distance x from the line of fall. In order to save himself he throws the bag horizontally (with respect to himself) in the direction opposite to the pond. Calculate the minimum horizontal velocity imparted to the bag so that the man lands in the water. If the man just succeeds to avoid the hard ground, where will the bag land?
Ans. 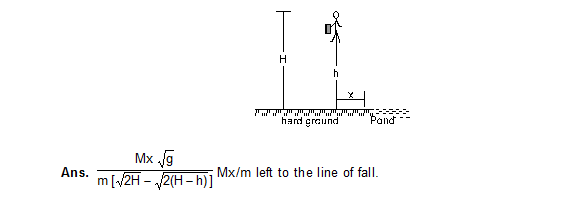
20. A ball of mass 50 g moving at a speed of 2.0 m/s strikes a plane surface at an angle of incidence 45°. The ball is reflected by the plane at equal angle of reflection with the same speed. Calculate (a) the magnitude of the change in momentum of the ball (b) the change in the magnitude of the momentum of the ball.
Ans. (a) 0.14 kg-m/s (b) zero
21. Light in certain cases may be considered as a stream of particles called photons. Each photon has a linear momentum h/l where h is the Planck’s constant and l is the wavelength of the light. A beam of light of wavelength l is incident on a plane mirror at an angle of incidence q. Calculate the change in the linear momentum of a photon as the beam is reflected by the mirror.

22. A block at rest explodes into three equal parts. Two parts starts moving along X and Y axes respectively with equal speeds of 10 m/s. Find the initial velocity of the third part.
![]()
23. Two fat astronauts each of mass 120 kg are travelling in a closed spaceship moving at a speed of 15 km/s in the outer space far removed from all other material objects. The total mass of the spaceship and its contents including the astronauts is 660 kg. If the astronauts do slimming exercise and thereby reduce their masses to 90 kg each, with what velocity will the spaceship move ?

Ans.15 km/s
24. During a heavy rain, hailstones of average size 1.0 cm in diameter fall with an average speed of 20 m/s. Suppose 2000 hailstones strike every square meter of a 10 m × 10 m roof perpendicularly in one second and assume that the hailstones do not rebound. Calculate the average force exerted by the falling hailstones on the roof. Density of a hailstones is 900 kg/m3.
Ans. 1900 N
25. A ball of mass m is dropped onto a floor from a certain height. The collision is perfectly elastic and the ball rebounds to the same height and again falls. Find the average force exerted by the ball on the floor during a long time interval.
Ans. mg
26. A rail rod car of mass M is at rest on frictionless rails when a man of mass m starts moving on the car towards the engine. If the car recoils with a speed v backward on the rails, with what velocity is the man approaching the engine?
Ans.
27. A gun is mounted on a railroad car. The mass of the car, the gun, the shells and the operator is 50 m where m is the mass of one shell. If the muzzle velocity of the shells is 200 m/s, what is the recoil speed of the car after the second shot? Neglect friction.
Ans. 200 m/s
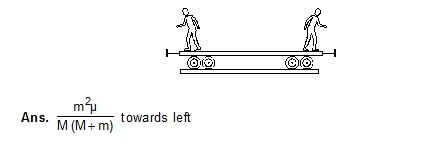
28. Two persons each of mass m are standing at the two extremes of a railroad car of mass M resting on a smooth track (figure). The person on left jumps to the left with a horizontal speed u with respect to the state of the car before the jump. Therefore, the other person jumps to the right, again with the same horizontal speed u with respect to the state of the car before his jumps. Find the velocity of the car after both the persons have jumped off.
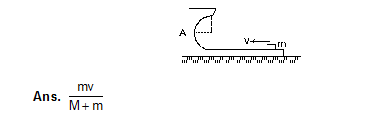
29. Figure shows a small block of mass m which is started with a speed v on the horizontal part of the bigger block of mass M placed on a horizontal floor. The curved part of the surface shown is semicircular. All the surfaces are frictionless. Find the speed of the bigger block when the smaller block reaches the point A of the surface.
30. In a typical Indian Bugghi (a luxury cart drawn by horses), a wooden plate is fixed on the rear on which one person can sit. A bugghi of mass 200 kg is moving at a speed of 10 km/hr. As it overtakes a school boy walking at a speed of 4 km/hr, the boy sits on the wooden plate. If the mass of the boy is 25 kg, what will be the new velocity of the bugghi?
Ans. 28/3 km/h
31. A ball of mass 0.50 kg moving at a speed of 5.0 m/s collides with another ball of mass 1.0 kg After the collision the ball stick together and remain motionless. What was the velocity of the 1.0 kg block before the collision?
Ans. 2.5 m/s opposite to the direction of motion of the first ball.
32. A 60 kg man skating with a speed of 10 m/s collides with a 40 kg skater at rest and they cling to each other. Find the loss of kinetic energy during the collision.
Ans. 1200 J
33. Consider a head - on collision between two particles of masses m1 and m2. The initial speeds of the particles are u1 and u2 in the same direction. The collision starts at t = 0 and the particles interact for a time interval Dt. During the collision, the speed of the first particle varies as

Find the speed of the second particle as a function of time during the collision.
Ans. 
34. A bullet of mass m moving at a speed v hits a ball of mass M kept at rest. A small part having mass m¢ breaks from the ball and sticks to the bullet. The remaining ball is found to move at a speed v1 in the direction of the bullet. Find the velocity of the bullet after the collision.
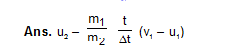
35. A ball of mass m moving at a speed v makes a head - on collision with an identical ball at rest. The kinetic energy of the balls after the collision is three fourths of the original. Find the coefficient of restitution.
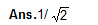
36. A block of mass 2.0 kg moving at 2.0 m/s collides head on with another block of equal mass kept at rest. (a) Find the maximum possible loss in kinetic energy due to the collision. (b) If the actual loss in kinetic energy is half of this maximum, find the coefficient of restitution.
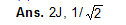
37. A particle of mass 100 g moving at an initial speed u collides with another particle of same mass kept initially at rest. If the total kinetic energy becomes 0.2 J after the collision, what could be the minimum and the maximum value of u.
![]()
38. Two friends A and B (each weighing 40 kg) are sitting on a frictionless platform some distance d apart. A rolls a ball of mass 4 kg on the platform towards B which B catches. Then B rolls the ball towards A and A catches it. The ball keeps on moving back and forth between A and B. The ball has a fixed speed of 5 m/s on the platform. (a) Find the speed of A after he rolls the ball for the first time. (b) Find the speed of A after he catches the ball for the first time. (c) Find the speeds of A and B after the ball has made 5 round trips and is held by A. (d) How many times can A roll the ball? (e) Where is the centre of mass of the system “ A + B + ball” at the end of the nth trip?
Ans. (a) 0.5 m/s (b)10/11 m/s (c) 50/11m/s, 5 m/s (d) 6 (e) 10/21 d
away from the initail position of A towards B.
39. A ball falls on the ground from a height of 2.0 m and rebounds upto a height of 1.5 m. Find the coefficient of restitution.
. 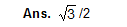
40. In a gamma decay process, the internal energy of a nucleus of mass M decreases, a gamma photon of energy E and linear momentum E/c is emitted and the nucleus recoils. Find the decrease in internal energy.
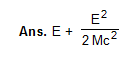
41. A block of mass 2.0 kg is moving on a frictionless horizontal surface with a velocity of
1.0 m/s (figure) towards another block of equal mass kept at rest. The spring constant of the spring fixed at one end is 100 N/m. Find the maximum compression of the spring.

Ans.10 cm
42. A bullet of mass 20 g travelling horizontally with a speed of 500 m/s passes through a wooden block of mass 10.0 kg initially at rest on a level surface. The bullet emerges with a speed of 100 m/s and the block slides 20 cm on the surface before coming to rest. Find the friction coefficient between the block and the surface (figure).

Ans. 0.16
43. A projectile is fired with a speed u at an angle q above a horizontal field. The coefficient of restitution of collision between the projectile and the field is e. How far from the starting point, does the projectile makes it second collision with the field?
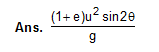
44. A ball falls on an inclined plane of inclination q from a height h above the point of impact and makes a perfectly elastic collision. Where will it hit the plane again?

45. Solve the previous problem if the coefficient of restitution is e.

Ans. 18.5 m along the incline
46. A block of mass 200 g is suspended through a vertical spring. The spring is stretched by
1.0 cm when the block is in equilibrium. A particle of mass 120 g is dropped on the block from a height of 45 cm. The particle sticks to the block after the impact. Find the maximum extension of the spring. Take g = 10 m/s2.
Ans. 6.1 cm
47. A bullet of mass 25 g is fired horizontally into a ballistic pendulum of mass 5.0 kg and gets embedded in it (figure). If the centre of the pendulum rises by a distance of 10 cm, find the speed of the bullet.
Ans. 280 m/s
48. A bullet of mass 20 g moving horizontally at a speed of 300 m/s is fired into a wooden block of mass 500 g suspended by a long string. The bullet crosses the block and emerges on the other side. If the centre of mass of the block rises through a height of 20.0 cm, find the speed of the bullet as it emerges from the block.
Ans. 250 m/s
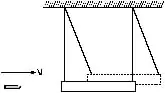
49. Two masses m1 and m2 are connected by a spring of spring constant k and are placed on a frictionless horizontal surface. Initially the spring is stretched through a distance x0 when the system is released from rest. Find the distance moved by the two masses before they again come to rest.
Ans. , 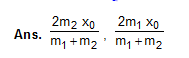
50. Two blocks of masses m1 and m2 are connected by spring of spring constant k (figure). The block of mass m2 is given a sharp impulse so that it acquires a velocity v0 towards right. Find (a) the velocity of the centre of mass, (b) the maximum elongation that the spring will suffer.

Ans. (a) (b)
51. Consider the situation of the previous problem. Suppose each of the blocks is pulled by a constant force F instead of any impulse. Find the maximum elongation that the spring will suffer and the distances moved by the two blocks in the process.

52. Consider the situation of the previous problem. Suppose the block of mass m1 is pulled by a constant force F1 and the other block is pulled by a constant force F2. Find the maximum elongation that the spring will suffer.

53. Consider a gravity-free hall in which an experimenter of mass 50 kg is resting on a 5 kg pillow, 8 ft above the floor of the hall. He pushes the pillow down so that it starts falling at a speed of 8 ft/s. The pillow makes a perfectly elastic collision with the floor, rebounds and reaches the experimenter’s head. Find the time elapsed in the process.
Ans. 2.22 s
54. The track shown in figure is frictionless. The block B of mass 2 m is lying at rest and the block A of mass m is placed along the track with some speed. The collision between A and B is perfectly elastic. With what velocity should the block A be started to get the sleeping man awakened ?
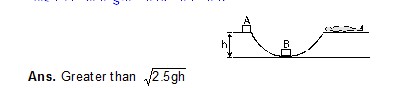
55. A bullet of mass 10g moving horizontally at a speed of 50m/s strikes a block of mass 490 g kept on a frictionless track as shown in figure. The bullet remains inside the block and the system proceeds towards the semicircular track of radius 0.2m. Where will the block strike the horizontal part after leaving the semicircular track?

Ans. At the junction of the straight and the curved parts
56. Two ball having masses m and 2m are fastened to two light strings of same length (figure). The other ends of the strings are fixed at O. The strings are kept in the same horizontal line and the system is released from rest. The collision between the balls is elastic. (a) Find the velocities of the balls just after their collision. (b) How high will the balls rise after the collision?
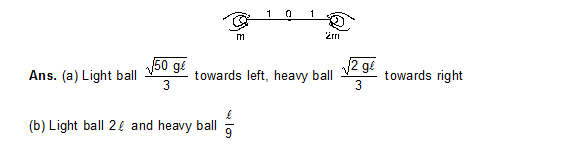
57. A uniform chain of mass M and length L is held vertically in such a way that its lower end just touches the horizontal floor. The chain in released from rest in this position. Any portion that strikes the floor comes to rest. Assuming that the chain does not form a heap on the floor, calculate the force exerted by it on the floor when a length x has reached the floor.
Ans. 3 Mgx/L
58. The blocks shown in figure have equal masses. The surface of A is smooth but that of B has a friction coefficient of 0.10 with the floor. Block A is moving at a speed of 10 m/s, towards B which is kept at rest. Find the distance travelled by B if (a) the collision is perfectly elastic and (b) the collision is perfectly inelastic. Take g = 10 m/s2.

Ans. (a) 50 m (b) 25 m
59. The friction coefficient between the horizontal surface and each of the blocks shown in figure is 0.20. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest. Take g = 10 m/s2.

Ans. 5 cm
60. A block of mass m is placed on a triangular block of mass M, which in turn is placed on a horizontal surface as shown in figure. Assuming frictionless surfaces find the velocity of the triangular block when the smaller block reaches the bottom end.

.
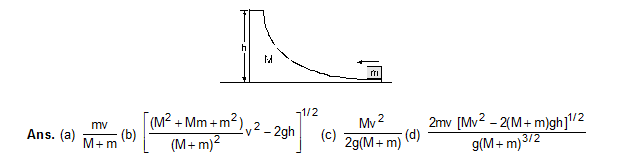
61. Figure shows a small body of mass m placed over a larger mass M whose surface is horizontal near the smaller mass and gradually curves to become vertical. The smaller mass is pushed on the longer one at a speed v and the system is left to itself. Assume that all the surfaces are frictionless. (a) Find the speed of the larger block when the smaller block is sliding on the vertical part. (b) Find the speed of the smaller mass when it breaks off the larger mass at height h. (c) Find the maximum height (from the ground) that the smaller mass ascends. (d) Show that the smaller mass will again land on the bigger one. Find the distance traversed by the bigger block during the time when the smaller block was in its flight under gravity.
62. A small block of superdense material has a mass of 3 × 1024 kg. It is situated at a height h (much smaller than earth’s radius) from where it falls on the earth’s surface. Find its speed when its height from the earth’s surface has reduced to h/2. The mass of earth is 6 × 1024 kg.
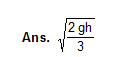
63. A body of mass m makes an elastic collision with another identical body at rest. Show that if the collision is not head-on, the bodies go at right angle to each other after the collision.
64. A small particle travelling with a velocity v collides elastically with a spherical body of equal mass and of radius r initially kept at rest. The centre of this spherical body is located a distance r(< r) away from the direction of motion of the particle (figure). Find the final velocities of the two particles.

[Hint : The force acts along the normal to the sphere through the contact. Treat the collision as one dimensional for this direction. In the tangential direction no force acts and the velocities do not change.]

1. (C) 2. (D) 3. (D) 4. (D)
5. (B) 6. (B) 7. (B) 8. (B)
9. (C) 10. (D) 11. (C) 12. (B)
13. (C) 14. (C) 15. (D) 16. (D)
17. (A) 18. (A) 19. (B)
1. None 2. (C, D) 3. (A, B) 4. (B, C)
5. (B, D) 6. (D) 7. (A, D) 8. (B, C, D)
9. (C, D) 10. (B, C) 11. All